Y – radiation, as mentioned, is fast, low cost, nondestructive, and easily automated. The principle used to measure density with y -radiation is simply to pass radiation through a material attenuating the radiation, in this case gamma particles. This principle is described by the Lambert-Beer law in eq. (1) (Tiitta, Olkkonen et al. 1996)
I(x) = Ioe-MPX (1)
where:
I(x) = radiation intensity after attenuation (counts/s.)
Io = unattenuated radiation intensity (counts/s.) ц = mass attenuation coefficient (cm2/g) p = density (g/cm3) x = absorber thickness (cm)
With known values of ц and x, and acknowledging the existence of some amount of background radiation, In, Equation (1) may be rearranged as (Tiitta, Olkkonen et al. 1996; Athanassiadis 2009)
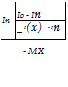
![]() (7)
(7)
The weakening of radiation depends on the thickness of a Y-rayed object and is also connected with the density of examined materials. Registering the interaction of low-energy Y-radiation with CM, we can get information about its structure and physical and chemical characteristics. Selecting composition and energy of radiation we can ensure the dominance of a particular type of interaction, to maximize the taken information of the controlled object.
Knowing the MAC of each element and element composition of analyzing object from the measured values of I and Io, we can determine the surface density P of object (Athanassiadis 1994):
![]() P = pl = —1—ln(^0/I)
P = pl = —1—ln(^0/I)
The knowledge of natural minerals’ physical parameters such as the mass attenuation coefficients M, the effective atomic number Zf is useful for understanding their physical properties.(Han, Demir et al. 2009).
It is important for densitometry of CM (Chudakov and Anshakov 1982), to define the effective parameters of y-radiation interaction with samples of complex chemical composition. (Plotnikov and Pschenicniji 1973) proposed replacement parameters conditionally substance consisting of one element, and use expressions:
W(ZejfE) = pA0 X A W(Z„E) (9)
Aeff і Ai
where q, – content of i-th-element $(Ze^£) — It is accordingly: doPhot (Zf E) – for a photo effect doCoh (Zeff, E) – for coherent scattering and doCom (Zeff, E) – for Compton scattering
In the case of incoherent scattering (Plotnikov and Pschenicniji 1973):
Zeff = 3 Yq. Zf (io)
and for coherent scattering (Plotnikov and Pschenicniji 1973):
Zff = ^БіЯ (11)



