Description of technical approach

Methodology and computational procedure is described as an integrated MS-PFA and probabilistic analysis capability. It is comprised of the following:

• determination of scatter and sensitivity of in-situ material properties and fabrication parameters (e. g., fiber tensile strength, and fiber volume ratio);

• reproducing the test measured scatter/distribution in lamina using MS-PFA, and probabilistic analysis;

• generate random samples using lamina level uncertainties;

• update scatter from simulation to match lamina CDF and PDF curves using Bayesian statistics followed by determination of allowables with the desired confidence levels;

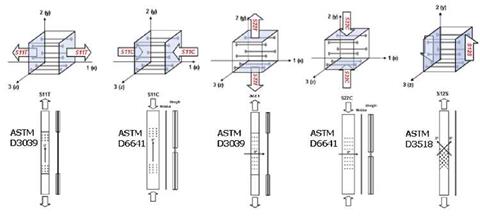
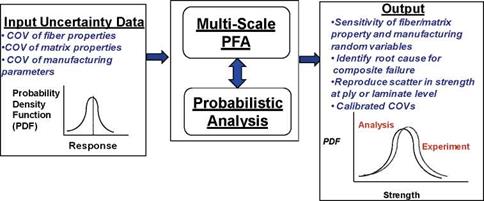
Scatter in strength data obtained from unidirectional lamina testing is used in predicting allowables for notched and un-notched laminates. The variability is generally caused by: (1) scatter in micro-scale mechanical properties of the fiber, matrix, and interface; (2) variability in composite manufacturing parameters; and (3) manufacturing defects such as void, waviness, and gaps. Lamina level testing is carried out to determine ply in-plane and out-of-plane mechanical properties. Table 2 lists the measured ply properties obtained from in-plane testing of composite specimens made from unidirectional laminates. The same table also lists the physical parameters influencing the measured ply response. For example, variation in ply longitudinal strength collected from test is a function of scatter of fiber strength and fiber content. MS-PFA [4] is used in conjunction with probabilistic methods [5] to reproduce scatter in strength obtained from lamina level testing. Figure 3 shows the lamina level testing performed per ASTM standards to generate in-plane ply properties. Figure 4 shows the process for reproducing the scatter from lamina level testing. Micro-scale random variables consisting of fiber and matrix mechanical properties combined with fabrication parameters are perturbed to reproduce the scatter from lamina level testing. This process results in a unique set of coefficient of variations for various random variables that can be used in random sampling of test specimens for higher order ASTM tests (laminate level).

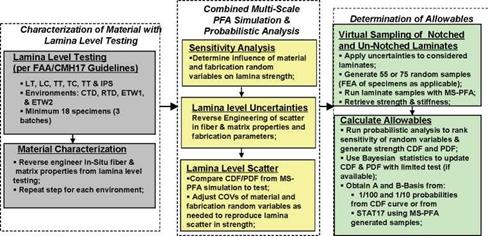
The use of lamina level uncertainties to predict allowables for laminate level building block tests is the added value of the work presented in this paper. The work reported in [6] confirms that lamina uncertainties are adequate for use in generation of scatter in laminate level response. The uncertainties causing scatter in strength of a composite laminate are: (1) variability in fiber and matrix properties and composite fabrication parameters; (2) manufacturing defects ("as designed" versus "as built" and "as is"); and (3) human error encountered during testing. A flow chart of the technical approach for determination of A – and B-basis allowables is presented in Figure 5. The basis for the computation is the reproduction of scatter in ply strength caused by variability of constituent material properties and manufacturing defects. The approach was validated by comparing its A – and B-basis predictions with values obtained from standard methods [1&2] using test data from robust or reduced sampling.